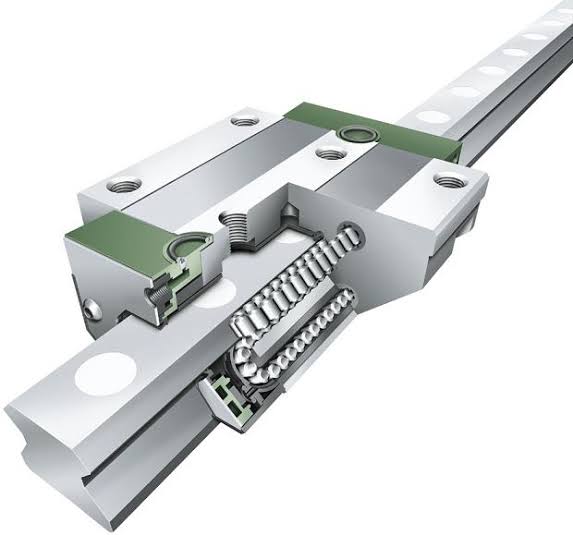
Linear guidance systems
Are indispensable for ensuring smooth, precise linear motion in many industrial applications. Whether in robotics, materials handling, automation or machine tool production, linear guides play an essential role in the smooth operation of a wide range of equipment. Linear